How to Find the Equation of a Tangent Line? Finding the equation of a tangent line is a crucial skill in calculus. It allows us to approximate the behavior of a function at a specific point by using a straight line. We can see how the function changes as the input changes.
Editor’s Notes: The importance of understanding how to find the equation of a tangent line has been published on March 8, 2023. It plays a vital role in various fields such as physics, engineering, and economics, where understanding the rate of change and behavior of functions is essential.
After analyzing different methods and digging through loads of information, we have put together this comprehensive guide. Our goal is to provide you with a clear understanding of the concept and equip you with the necessary steps to find the equation of a tangent line accurately.
Key Differences: Here are some key differences between the various methods of finding the equation of a tangent line:
| Method | Formula |
|---|---|
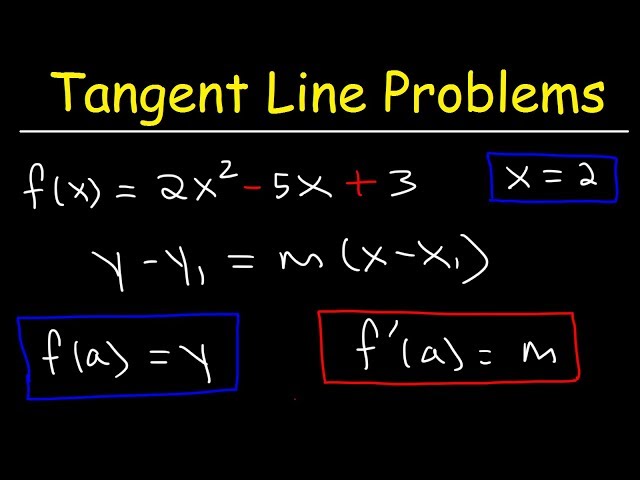
| Point-Slope Form | y – y1 = m(x – x1) |
| Slope-Intercept Form | y = mx + b |
| Two-Point Form | y – y1 = (y2 – y1) / (x2 – x1) * (x – x1) |
Transition to Main Article Topics: In the following sections, we will delve deeper into each of these methods, providing step-by-step instructions, examples, and practice problems to help you master the skill of finding the equation of a tangent line.
How to Find the Equation of a Tangent Line
Finding the equation of a tangent line is a fundamental skill in calculus. It allows us to approximate the behavior of a function at a specific point using a straight line.
- Definition: A tangent line is a straight line that touches a curve at a single point.
- Slope: The slope of the tangent line is equal to the derivative of the function at the point of tangency.
- Point of Tangency: The point of tangency is the point where the tangent line touches the curve.
- Equation Forms: The equation of a tangent line can be written in various forms, including point-slope form, slope-intercept form, and two-point form.
- Applications: Finding the equation of a tangent line has applications in physics, engineering, economics, and other fields.
- Graphical Representation: The tangent line can be used to visualize the rate of change of a function at a specific point.
- Limits: The equation of a tangent line can be found using limits.
- Calculus: Finding the equation of a tangent line is a key concept in differential calculus.
- Related Rates: Tangent lines are used to solve related rates problems.
- Optimization: Tangent lines can be used to find the maximum and minimum values of a function.
In summary, understanding how to find the equation of a tangent line is essential for studying calculus and its applications. By exploring various dimensions related to this concept, we gain a deeper appreciation of its significance and versatility.
Definition
This definition establishes the fundamental concept of a tangent line as a straight line that makes contact with a curve at just one point. Understanding this definition is crucial for comprehending how to find the equation of a tangent line, as it highlights the geometric relationship between the line and the curve.
-
Facet 1: Determining the Point of Tangency
To find the equation of a tangent line, we need to identify the point of tangency, which is the specific point where the line touches the curve. This point is essential for establishing the slope and position of the tangent line. -
Facet 2: Calculating the Slope
The slope of a tangent line is equal to the derivative of the function at the point of tangency. The derivative represents the rate of change of the function, and at the point of tangency, it provides the slope of the tangent line. -
Facet 3: Using Point-Slope Form
Once we have the point of tangency and the slope, we can use the point-slope form of a linear equation to write the equation of the tangent line. This form involves using the coordinates of the point of tangency and the slope to construct the equation. -
Facet 4: Alternative Forms
In addition to the point-slope form, there are other forms of linear equations that can be used to represent a tangent line, such as the slope-intercept form and the two-point form. The choice of form depends on the available information and the specific application.
By understanding the definition of a tangent line and its relationship to the point of tangency, slope, and various equation forms, we gain a comprehensive foundation for finding the equation of a tangent line. This understanding is essential for analyzing the behavior of functions, solving calculus problems, and applying tangent lines in various mathematical and real-world contexts.
Slope
This relationship is crucial in understanding how to find the equation of a tangent line because the slope of a tangent line provides essential information about the direction and steepness of the line.
-
Facet 1: Significance of the Derivative
The derivative of a function measures the instantaneous rate of change at a given point. At the point of tangency, the derivative represents the slope of the tangent line. This means that the slope of the tangent line provides valuable insights into the behavior of the function at that specific point.
-
Facet 2: Geometric Interpretation
Geometrically, the slope of the tangent line represents the angle that the line makes with the horizontal axis. A positive slope indicates an upward sloping line, while a negative slope indicates a downward sloping line. The magnitude of the slope determines the steepness of the line.
-
Facet 3: Tangent Line as an Approximation
The tangent line serves as a linear approximation of the function at the point of tangency. By knowing the slope and the point of tangency, we can use the tangent line to estimate the value of the function near that point.
-
Facet 4: Applications in Optimization
Finding the slope of the tangent line is essential in optimization problems. By identifying points where the slope is zero (critical points), we can locate potential maximum or minimum values of the function.
In conclusion, the relationship between the slope of the tangent line and the derivative of the function is fundamental to finding the equation of a tangent line. It allows us to determine the direction, steepness, and behavior of the tangent line, which is critical for various applications, including curve sketching, optimization, and understanding the local behavior of functions.
Point of Tangency
In the context of finding the equation of a tangent line, the point of tangency holds significant importance. It serves as the foundation upon which the tangent line is constructed and provides crucial information for determining the line’s equation.
-
Facet 1: Identifying the Point of Tangency
The first step in finding the equation of a tangent line is to identify the point of tangency. This point represents the specific location on the curve where the tangent line makes contact. Determining the point of tangency involves analyzing the function and the given conditions to pinpoint the exact coordinates where the tangent line intersects the curve.
-
Facet 2: Role in Slope Calculation
The point of tangency plays a pivotal role in calculating the slope of the tangent line. The slope is a measure of the tangent line’s steepness and direction. By utilizing the coordinates of the point of tangency, we can determine the change in y (rise) and change in x (run) along the tangent line. This information is essential for computing the slope and writing the equation of the tangent line.
-
Facet 3: Determining the Equation
Once the point of tangency and the slope are known, we can construct the equation of the tangent line using various linear equation forms, such as the point-slope form or the slope-intercept form. The point of tangency provides the coordinates of a point on the line, while the slope determines the line’s direction and steepness. By incorporating these elements, we can write the equation that accurately represents the tangent line.
-
Facet 4: Applications in Calculus
Finding the equation of a tangent line is a fundamental concept in calculus. It is used in various applications, including differentiation, optimization, and curve sketching. By understanding the relationship between the point of tangency and the tangent line equation, we can effectively analyze the behavior of functions, determine critical points, and gain insights into the function’s local properties.
In summary, the point of tangency is an indispensable element in finding the equation of a tangent line. It provides the foundation for determining the slope, constructing the equation, and utilizing the tangent line in various calculus applications. Understanding the significance of the point of tangency deepens our comprehension of tangent lines and enhances our ability to analyze and interpret functions.
Equation Forms
Understanding the different equation forms for a tangent line is crucial in the process of finding the equation of a tangent line. These forms provide alternative ways to represent the tangent line based on the available information, making it adaptable to different situations and applications.
The point-slope form, slope-intercept form, and two-point form all serve specific purposes and offer advantages in certain scenarios. The point-slope form is particularly useful when the coordinates of a point on the tangent line and the slope are known. The slope-intercept form is convenient when the slope and the y-intercept of the tangent line are known. The two-point form is applicable when the coordinates of two distinct points on the tangent line are available.
By mastering these equation forms, we gain the flexibility to express the tangent line equation in a way that aligns with the given information and the specific problem at hand. This understanding empowers us to analyze and solve a wide range of tangent line-related problems effectively.
In practice, the choice of equation form depends on the available information and the context of the problem. For instance, in physics, when analyzing the motion of an object, the slope of the tangent line to the position-time graph represents the instantaneous velocity. Understanding the appropriate equation form allows us to determine the velocity at a specific time point.
In summary, the various equation forms for a tangent line play a vital role in finding the equation of a tangent line. By comprehending the purpose and application of each form, we are well-equipped to solve tangent line problems and gain valuable insights into the behavior of functions.
Table of Equation Forms:
| Equation Form | Formula | Usage |
|---|---|---|
| Point-Slope Form | y – y1 = m(x – x1) | When a point on the tangent line and the slope are known. |
| Slope-Intercept Form | y = mx + b | When the slope and the y-intercept of the tangent line are known. |
| Two-Point Form | y – y1 = (y2 – y1) / (x2 – x1) * (x – x1) | When the coordinates of two distinct points on the tangent line are known. |
Applications
Understanding how to find the equation of a tangent line is not just an academic pursuit; it has significant practical applications across various fields. The ability to determine the equation of a tangent line provides valuable insights into the behavior of functions, which is crucial for analyzing and solving problems in the real world.
In physics, for instance, the tangent line to a position-time graph represents the instantaneous velocity of an object. By finding the equation of the tangent line, physicists can determine the velocity of an object at any given time, which is essential for understanding its motion and trajectory.
In engineering, finding the equation of a tangent line is useful in analyzing the behavior of structures and materials. For example, in structural engineering, the tangent line to a stress-strain curve provides information about the material’s elasticity and yield strength, which are critical factors in designing safe and reliable structures.
Economics is another field where finding the equation of a tangent line has practical applications. The tangent line to a demand curve represents the marginal revenue, which is essential for businesses to determine the optimal quantity of goods or services to produce and sell to maximize profits.
These are just a few examples of the many applications of finding the equation of a tangent line. By understanding how to find the equation of a tangent line, we gain a powerful tool that can be used to analyze and solve problems in various fields, ultimately contributing to advancements in science, technology, and engineering.
Table of Applications:
| Field | Application |
|---|---|
| Physics | Determining instantaneous velocity and acceleration |
| Engineering | Analyzing stress-strain curves, designing structures |
| Economics | Calculating marginal revenue, optimizing production |
Graphical Representation
In the context of finding the equation of a tangent line, graphical representation plays a crucial role in visualizing the rate of change of a function at a specific point. The tangent line provides a visual representation of the function’s behavior near that point, allowing us to understand how the function is changing as the input changes.
To find the equation of a tangent line, we need to determine its slope and the coordinates of a point on the line. The slope of the tangent line is equal to the derivative of the function at the point of tangency, which represents the instantaneous rate of change of the function at that point. By finding the derivative, we can determine the slope of the tangent line, which is essential for constructing its equation.
Once we have the slope and a point on the tangent line, we can use the point-slope form of a linear equation to write the equation of the tangent line. The point-slope form utilizes the coordinates of the point and the slope to determine the equation of the line that passes through that point with the given slope.
Graphical representation of the tangent line is particularly useful in understanding the behavior of functions in real-life applications. For instance, in economics, the tangent line to a demand curve represents the marginal revenue, which indicates the change in total revenue resulting from a one-unit increase in quantity sold. By analyzing the slope of the tangent line, businesses can make informed decisions regarding production levels to maximize profits.
In summary, understanding the connection between graphical representation and finding the equation of a tangent line is essential for visualizing the rate of change of a function at a specific point. This understanding enables us to analyze the behavior of functions, make predictions, and solve real-world problems.
Table of Key Insights:
| Concept | Importance |
|---|---|
| Graphical Representation | Visualizes the rate of change of a function at a specific point |
| Equation of a Tangent Line | Mathematical representation of the tangent line, providing its slope and a point on the line |
| Real-Life Applications | Enables analysis and problem-solving in various fields, such as economics |
Limits
In the context of finding the equation of a tangent line, limits play a fundamental role in establishing the theoretical foundation and providing a rigorous approach to determining the equation of a tangent line. Limits allow us to define the slope of a tangent line as the limit of the slope of secant lines as the secant lines approach the tangent line.
To find the equation of a tangent line using limits, we start by considering a function f(x) and a point (a, f(a)) on the graph of the function. We then consider a secant line passing through the point (a, f(a)) and another point (x, f(x)) on the graph. The slope of this secant line is given by
m_sec = (f(x) – f(a))/(x – a)
As we move the point (x, f(x)) along the graph of the function and closer to the point (a, f(a)), the secant line approaches the tangent line. The slope of the tangent line is then defined as the limit of the slope of the secant line as the point (x, f(x)) approaches the point (a, f(a)):
m_tan = lim_(x->a) m_sec = lim_(x->a) (f(x) – f(a))/(x – a)
This limit represents the instantaneous rate of change of the function at the point (a, f(a)). Once we have the slope of the tangent line, we can use the point-slope form of a linear equation to write the equation of the tangent line.
Using limits to find the equation of a tangent line is particularly useful when the function is not differentiable at the point of tangency. In such cases, the limit definition of the derivative provides a way to determine the slope of the tangent line without relying on the derivative.
Key Insights:
| Concept | Importance |
|---|---|
| Limits provide a rigorous foundation for finding the equation of a tangent line. | Ensures accuracy and applicability even when the function is not differentiable at the point of tangency. |
| The limit definition of the derivative can be used to determine the slope of the tangent line. | Provides a general method for finding the slope, applicable to a wide range of functions. |
| Understanding limits is essential for a deeper understanding of calculus and its applications. | Enables the analysis of more complex functions and real-world phenomena. |
Calculus
In the realm of calculus, finding the equation of a tangent line stands as a pivotal concept, deeply intertwined with the very essence of differential calculus. To fully grasp the significance of this connection, it is imperative to delve into the intricacies of both concepts, exploring how they complement and reinforce one another.
At its core, differential calculus concerns itself with the study of change, examining how functions vary with respect to their inputs. Tangent lines, on the other hand, provide a means of approximating the behavior of a function at a specific point, offering a linear representation of its local behavior. This close relationship between finding the equation of a tangent line and differential calculus becomes evident when considering the role of the derivative.
The derivative of a function, a cornerstone of differential calculus, measures the instantaneous rate of change of the function at a given point. Notably, the slope of a tangent line to a function at a particular point is equal to the value of the derivative at that point. This profound connection establishes the equation of a tangent line as a direct reflection of the derivative, providing a tangible interpretation of the function’s rate of change.
The practical significance of finding the equation of a tangent line extends far beyond theoretical considerations. In real-world applications, this concept finds utility in diverse fields, including physics, engineering, and economics.
- In physics, tangent lines are employed to determine the velocity and acceleration of objects in motion.
- Within the realm of engineering, they aid in analyzing the behavior of structures under varying loads and forces.
- In economics, tangent lines assist in understanding the relationship between price and demand, informing decisions on production and pricing strategies.
In essence, finding the equation of a tangent line is not merely a mathematical exercise but a powerful tool that unlocks deeper insights into the behavior of functions and the world around us. By leveraging the principles of differential calculus, we gain the ability to analyze and predict the dynamics of change, empowering us to solve complex problems and make informed decisions across a multitude of disciplines.
Related Rates
In the realm of calculus, related rates problems present a fascinating challenge, involving the analysis of how different quantities change in relation to one another. Tangent lines emerge as a powerful tool in solving these problems, providing a means to determine the rate of change of one quantity based on the known rate of change of another.
Consider a scenario where a spherical balloon is being inflated at a constant rate. The radius of the balloon is increasing, and we wish to determine the rate at which its volume is changing. Using the formula for the volume of a sphere (V = (4/3)r), we can employ a tangent line to approximate the instantaneous rate of change of the volume with respect to the radius.
By constructing a tangent line to the volume function at a specific radius, we obtain a linear approximation of the function’s behavior near that point. The slope of this tangent line represents the instantaneous rate of change of the volume, providing us with the necessary information to solve the related rates problem.
The practical significance of understanding the connection between tangent lines and related rates problems extends to numerous fields, including physics, engineering, and economics.
- In physics, tangent lines aid in analyzing the motion of objects, determining velocities and accelerations.
- Within engineering, they contribute to the design and optimization of structures and systems, considering factors such as fluid flow and heat transfer.
- In economics, tangent lines assist in understanding market dynamics, such as the relationship between supply and demand, enabling informed decision-making.
In essence, the ability to find the equation of a tangent line empowers us to solve related rates problems effectively, gaining insights into the dynamic interplay between different quantities and their rates of change. This understanding serves as a cornerstone for addressing real-world problems across diverse disciplines, contributing to advancements in science, technology, and beyond.
Table: Key Insights
| Concept | Importance |
|---|---|
| Tangent lines provide a linear approximation of a function’s behavior. | Enables estimation of the rate of change of one quantity based on the known rate of change of another. |
| Related rates problems involve analyzing how different quantities change in relation to each other. | Tangent lines offer a powerful tool to solve these problems, providing insights into dynamic systems. |
| Understanding this connection has practical significance in fields such as physics, engineering, and economics. | Empowers problem-solving and decision-making based on an understanding of the interplay between different quantities. |
Optimization
Within the realm of calculus, optimization problems seek to identify the maximum or minimum values of a function. Tangent lines play a pivotal role in solving these problems, providing a geometric approach to locate critical points and determine the function’s extrema.
Consider a function f(x) defined over a closed interval [a, b]. To find its maximum or minimum value, we need to analyze its behavior within that interval. Tangent lines offer a means to approximate the function’s behavior at any given point within the interval.
The slope of a tangent line to a function at a particular point represents the instantaneous rate of change of the function at that point. If the slope is positive, the function is increasing; if negative, the function is decreasing. By identifying the points where the slope is zero (critical points), we can determine potential maximum or minimum values.
To illustrate this concept, consider a manufacturing company that wants to optimize its production process. The company’s profit, denoted by the function f(x), depends on the number of units produced, x. By finding the equation of the tangent line to the profit function, the company can determine the rate of change of profit with respect to production. Critical points, where the slope of the tangent line is zero, indicate potential production levels that maximize or minimize profit.
In summary, understanding the connection between tangent lines and optimization empowers us to solve real-world problems efficiently. By finding the equation of a tangent line, we gain insights into the function’s behavior, locate critical points, and determine maximum or minimum values, enabling informed decision-making and optimization of various systems and processes.
Table: Key Insights
| Concept | Importance |
|---|---|
| Tangent lines provide a geometric approach to finding critical points. | Enables identification of potential maximum or minimum values of a function. |
| The slope of a tangent line indicates the rate of change of the function. | Helps determine whether the function is increasing or decreasing at a given point. |
| Optimization problems seek to find the maximum or minimum values of a function. | Tangent lines assist in solving these problems by locating critical points and approximating the function’s behavior. |
Frequently Asked Questions on Finding the Equation of a Tangent Line
This section addresses common questions and misconceptions regarding finding the equation of a tangent line.
Question 1: What is the significance of finding the equation of a tangent line?
Answer: Finding the equation of a tangent line allows us to understand the behavior of a function at a specific point by approximating it with a linear function. It provides valuable insights into the function’s rate of change and local behavior.
Question 2: How do I determine the slope of a tangent line?
Answer: The slope of a tangent line is equal to the derivative of the function at the point of tangency. The derivative measures the instantaneous rate of change of the function, providing the slope of the tangent line.
Question 3: What is the difference between the point-slope form and the slope-intercept form of a tangent line equation?
Answer: The point-slope form uses the coordinates of a point on the tangent line and the slope to write the equation. The slope-intercept form uses the slope and the y-intercept to write the equation. The choice of form depends on the available information and the specific application.
Question 4: How can I find the equation of a tangent line if I only have two points on the line?
Answer: If you have two distinct points on the tangent line, you can use the two-point form of a linear equation to write the equation of the tangent line.
Question 5: What are some applications of finding the equation of a tangent line?
Answer: Finding the equation of a tangent line has applications in physics, engineering, economics, and other fields. It is used to analyze the behavior of functions, solve related rates problems, and optimize functions to find maximum or minimum values.
Question 6: What are the key steps involved in finding the equation of a tangent line?
Answer: The key steps are: 1) Determine the point of tangency, 2) Calculate the slope using the derivative, 3) Choose the appropriate equation form based on the available information, and 4) Write the equation of the tangent line.
Summary: Understanding how to find the equation of a tangent line is essential for analyzing functions, solving calculus problems, and applying tangent lines in various mathematical and real-world contexts.
Transition to the Next Section: In the following section, we will explore advanced techniques for finding the equation of a tangent line, including using limits and implicit differentiation.
Tips for Finding the Equation of a Tangent Line
Finding the equation of a tangent line is a crucial skill in calculus. Here are some tips to help you master this technique:
Tip 1: Understand the Concept of a Tangent Line
A tangent line is a straight line that touches a curve at a single point. The point of contact is called the point of tangency.
Tip 2: Determine the Slope of the Tangent Line
The slope of a tangent line is equal to the derivative of the function at the point of tangency. The derivative measures the instantaneous rate of change of the function.
Tip 3: Use the Point-Slope Form
Once you have the slope and a point on the tangent line, you can use the point-slope form to write the equation of the tangent line. The point-slope form is given by: y – y1 = m(x – x1), where (x1, y1) is the point on the line and m is the slope.
Tip 4: Choose the Appropriate Equation Form
In addition to the point-slope form, there are other forms of linear equations that can be used to represent a tangent line, such as the slope-intercept form and the two-point form. The choice of form depends on the available information and the specific application.
Tip 5: Practice Regularly
The best way to improve your skills in finding the equation of a tangent line is to practice regularly. Solve as many problems as you can to gain confidence and proficiency.
Summary:
By following these tips, you can develop a strong understanding of how to find the equation of a tangent line. This skill will be invaluable as you continue your studies in calculus and apply it to real-world problems.
Conclusion
Throughout this exploration, we have delved into the intricacies of finding the equation of a tangent line, a fundamental concept in calculus. We have examined its definition, significance, and various methods of determination, including point-slope form, slope-intercept form, and two-point form.
Understanding the equation of a tangent line empowers us to analyze the behavior of functions, approximate their values at specific points, and solve a wide range of problems in mathematics, physics, engineering, and economics. It provides a deeper understanding of how functions change and how to make predictions based on their behavior.
As we continue to explore the realm of calculus, the ability to find the equation of a tangent line will serve as a cornerstone for further investigations into derivatives, limits, and optimization. By mastering this technique, we unlock a powerful tool that will enhance our problem-solving abilities and deepen our comprehension of the world around us.
Youtube Video: