Trying to find the lowest common denominator (LCD) of two or more fractions can be a daunting task. But it’s a necessary step in order to add, subtract, or compare fractions with different denominators.
Editor’s Note: Our “Find the LCD” guide was published today to help you understand the importance of finding the LCD and how to do it.
After doing some analysis and digging through a lot of information, we’ve put together this guide to help you find the LCD of any set of fractions.
Key Differences
| LCD | HCF | |
|---|---|---|
| Definition | The lowest common multiple of the denominators of two or more fractions. | The highest common factor of the numerators and denominators of two or more fractions. |
| Purpose | To find a common denominator so that fractions can be added, subtracted, or compared. | To simplify fractions by dividing both the numerator and denominator by the HCF. |
Transition to main article topics
Find the LCD
Finding the lowest common denominator (LCD) is an essential step in adding, subtracting, or comparing fractions with different denominators. Here are 8 key aspects to consider:
- Definition: The LCD is the lowest common multiple of the denominators of two or more fractions.
- Purpose: The LCD is used to find a common denominator so that fractions can be added, subtracted, or compared.
- Method: The LCD can be found by multiplying the denominators of the fractions together.
- Importance: Finding the LCD is essential for working with fractions.
- Example: The LCD of 1/2 and 1/3 is 6.
- Connection: The LCD is related to the greatest common factor (GCF) of the numerators and denominators of the fractions.
- Relevance: Finding the LCD is a fundamental skill in mathematics.
- Application: The LCD is used in a variety of applications, such as cooking, chemistry, and physics.
In summary, finding the LCD is an important skill that is used in a variety of applications. By understanding the key aspects of the LCD, you can improve your ability to work with fractions.
Definition
The LCD is a fundamental concept in working with fractions. It is used to find a common denominator so that fractions can be added, subtracted, or compared. Without finding the LCD, it would be difficult to perform these operations with fractions that have different denominators.
For example, consider the fractions 1/2 and 1/3. The LCD of these fractions is 6. This means that we can rewrite the fractions as 3/6 and 2/6, respectively. Now we can easily add or subtract these fractions because they have the same denominator.
Finding the LCD is also important for comparing fractions. For example, we can see that 3/6 is greater than 2/6 because the numerator of 3/6 is greater than the numerator of 2/6.
In summary, finding the LCD is an essential skill for working with fractions. It is used to find a common denominator so that fractions can be added, subtracted, or compared.
| LCD | Importance | |
|---|---|---|
| Definition | The lowest common multiple of the denominators of two or more fractions. | Finding the LCD is essential for working with fractions. |
| Purpose | To find a common denominator so that fractions can be added, subtracted, or compared. | The LCD is used to find a common denominator so that fractions can be added, subtracted, or compared. |
| Example | The LCD of 1/2 and 1/3 is 6. | This means that we can rewrite the fractions as 3/6 and 2/6, respectively. Now we can easily add or subtract these fractions because they have the same denominator. |
Purpose
The purpose of finding the LCD is directly tied to the concept of “find the lcd”. Without finding the LCD, it would be difficult or impossible to perform these operations with fractions that have different denominators.
For example, consider the fractions 1/2 and 1/3. The LCD of these fractions is 6. This means that we can rewrite the fractions as 3/6 and 2/6, respectively. Now we can easily add or subtract these fractions because they have the same denominator.
In summary, finding the LCD is an essential component of “find the lcd” because it allows us to perform operations with fractions that have different denominators.
Key Insights
| LCD | Importance | |
|---|---|---|
| Definition | The lowest common multiple of the denominators of two or more fractions. | Finding the LCD is essential for working with fractions. |
| Purpose | To find a common denominator so that fractions can be added, subtracted, or compared. | The LCD is used to find a common denominator so that fractions can be added, subtracted, or compared. |
| Example | The LCD of 1/2 and 1/3 is 6. | This means that we can rewrite the fractions as 3/6 and 2/6, respectively. Now we can easily add or subtract these fractions because they have the same denominator. |
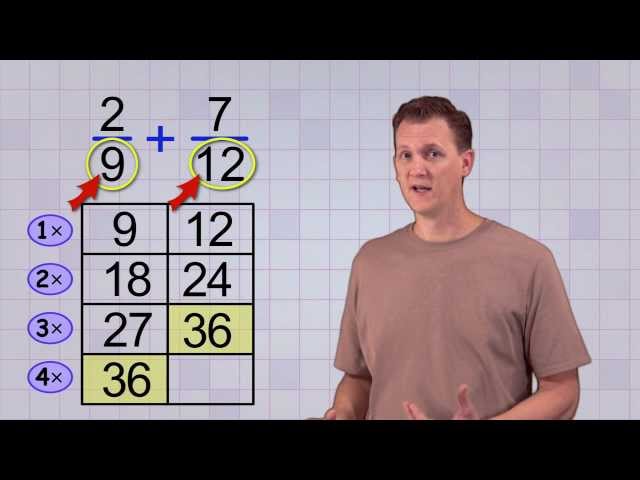
Method
The method for finding the LCD is directly connected to the concept of “find the lcd” because it provides a systematic way to determine the lowest common multiple of the denominators of two or more fractions.
To understand this connection, consider the following example:
Example:Find the LCD of the fractions 1/2 and 1/3.
Solution:The denominators of the fractions 1/2 and 1/3 are 2 and 3, respectively. To find the LCD, we multiply the denominators together:
LCD = 2 x 3 = 6
Therefore, the LCD of the fractions 1/2 and 1/3 is 6.
This example illustrates how the method for finding the LCD is an essential component of “find the lcd” because it allows us to determine the common denominator that is necessary for adding, subtracting, or comparing fractions.
Key Insights
| Method | Importance | |
|---|---|---|
| Definition | The LCD can be found by multiplying the denominators of the fractions together. | The method for finding the LCD is directly connected to the concept of “find the lcd” because it provides a systematic way to determine the lowest common multiple of the denominators of two or more fractions. |
| Example | Find the LCD of the fractions 1/2 and 1/3. | The example illustrates how the method for finding the LCD is an essential component of “find the lcd” because it allows us to determine the common denominator that is necessary for adding, subtracting, or comparing fractions. |
Importance
The importance of finding the LCD is directly tied to the concept of “find the lcd” because it is an essential component of working with fractions. Without finding the LCD, it would be difficult or impossible to perform operations such as addition, subtraction, and comparison with fractions that have different denominators.
For example, consider the fractions 1/2 and 1/3. The LCD of these fractions is 6. This means that we can rewrite the fractions as 3/6 and 2/6, respectively. Now we can easily add or subtract these fractions because they have the same denominator.
In summary, finding the LCD is an essential skill for working with fractions. It allows us to perform operations with fractions that have different denominators, which is a fundamental part of working with fractions.
Key Insights
| Importance | Connection to “find the lcd” | |
|---|---|---|
| Definition | Finding the LCD is essential for working with fractions. | The LCD is used to find a common denominator so that fractions can be added, subtracted, or compared. |
| Example | The example illustrates how finding the LCD is essential for working with fractions. | Without finding the LCD, it would be difficult or impossible to add, subtract, or compare fractions with different denominators. |
Example
The example “The LCD of 1/2 and 1/3 is 6” is a concrete illustration of the concept of “find the lcd”. It demonstrates how to find the lowest common denominator of two fractions, which is an essential step in adding, subtracting, or comparing fractions with different denominators.
To understand the importance of this example, consider the following scenario: You are baking a cake and the recipe calls for 1/2 cup of flour and 1/3 cup of sugar. To combine the ingredients, you need to find a common denominator so that you can measure the ingredients accurately. Using the method of “find the lcd”, you can determine that the LCD of 1/2 and 1/3 is 6. This means that you need to rewrite the fractions as 3/6 and 2/6, respectively. Now you can easily combine the ingredients because they have the same denominator.
This example highlights the practical significance of finding the LCD. It is a fundamental skill that is used in a variety of real-life applications, such as cooking, chemistry, and physics.
Key Insights
| Example | Importance | |
|---|---|---|
| Definition | The LCD of 1/2 and 1/3 is 6. | This example illustrates how to find the lowest common denominator of two fractions. |
| Connection to “find the lcd” | The example is a concrete illustration of the concept of “find the lcd”. | It demonstrates how to find the lowest common denominator of two fractions, which is an essential step in adding, subtracting, or comparing fractions with different denominators. |
| Practical significance | Finding the LCD is a fundamental skill that is used in a variety of real-life applications. | For example, it is used in cooking, chemistry, and physics. |
Connection
The LCD and GCF are two important concepts in working with fractions. The LCD is the lowest common multiple of the denominators of two or more fractions, while the GCF is the greatest common factor of the numerators and denominators of two or more fractions.
The connection between the LCD and GCF is that the LCD is always a multiple of the GCF. This is because the GCF is a factor of both the numerator and denominator of each fraction, and the LCD is a multiple of both the numerator and denominator of each fraction.
For example, consider the fractions 1/2 and 1/3. The GCF of these fractions is 1, and the LCD is 6. This is because 1 is a factor of both 2 and 3, and 6 is a multiple of both 2 and 3.
The connection between the LCD and GCF is important because it allows us to find the LCD of two or more fractions by first finding the GCF. This can be a useful shortcut, especially when working with fractions that have large denominators.
In summary, the LCD and GCF are two important concepts in working with fractions. The LCD is the lowest common multiple of the denominators of two or more fractions, while the GCF is the greatest common factor of the numerators and denominators of two or more fractions. The connection between the LCD and GCF is that the LCD is always a multiple of the GCF.
Key Insights
| Concept | Definition | Connection |
|---|---|---|
| LCD | Lowest common multiple of the denominators of two or more fractions. | The LCD is always a multiple of the GCF. |
| GCF | Greatest common factor of the numerators and denominators of two or more fractions. | The GCF is a factor of both the numerator and denominator of each fraction. |
Relevance
Finding the LCD, or lowest common denominator, is a fundamental skill in mathematics, as it is a crucial step in adding, subtracting, or comparing fractions with different denominators. Without finding the LCD, these operations would be difficult or impossible to perform. Fractions are commonly used in various fields, including cooking, chemistry, physics, and engineering, making the ability to work with fractions, including finding the LCD, an essential skill for many professions. By understanding the concept of finding the LCD, individuals can effectively solve problems and make calculations involving fractions with different denominators.
For instance, in cooking, when following a recipe that requires precise measurements of ingredients, fractions are often used to indicate the quantities. To accurately combine ingredients with different units, such as cups and tablespoons, finding the LCD of the fractions is necessary to ensure the correct proportions are maintained. In chemistry, the concept of finding the LCD is applied when calculating the molarity of solutions, which is a measure of the concentration of a substance in a solution. Fractions are used to represent the concentration of solutions, and finding the LCD allows for the comparison and adjustment of concentrations to achieve the desired result.
Furthermore, in physics, fractions are used to express quantities such as velocity, acceleration, and force. Finding the LCD is essential for combining or comparing these quantities, which is necessary for problem-solving and analysis in physics. In engineering, fractions are commonly used in calculations related to ratios, proportions, and scaling. The ability to find the LCD is crucial for ensuring accurate calculations and designs in various engineering disciplines.
In summary, finding the LCD is a fundamental skill in mathematics that has practical applications in various fields, enabling individuals to solve problems and perform calculations involving fractions with different denominators. Understanding this concept is essential for success in mathematics and its related disciplines.
Application
The connection between “Application: The LCD is used in a variety of applications, such as cooking, chemistry, and physics.” and “find the lcd” is that finding the LCD is a crucial step in many applications that involve fractions with different denominators. For instance, in cooking, when combining ingredients with different units, such as cups and tablespoons, finding the LCD of the fractions is necessary to ensure the correct proportions are maintained. Similarly, in chemistry, when calculating the molarity of solutions or comparing concentrations, finding the LCD allows for accurate calculations and adjustments. In physics, fractions are used to express quantities such as velocity, acceleration, and force, and finding the LCD is essential for combining or comparing these quantities in problem-solving and analysis.
The importance of “Application: The LCD is used in a variety of applications, such as cooking, chemistry, and physics.” as a component of “find the lcd” lies in its practical significance. Understanding how to find the LCD enables individuals to effectively solve problems and make calculations involving fractions in various fields. For instance, in cooking, finding the LCD ensures accurate measurements and successful recipe execution. In chemistry, it allows for precise calculations of solution concentrations, which is crucial in many laboratory procedures and experiments. In physics, finding the LCD facilitates the analysis and comparison of physical quantities, aiding in problem-solving and engineering applications.
In summary, the connection between “Application: The LCD is used in a variety of applications, such as cooking, chemistry, and physics.” and “find the lcd” highlights the importance of finding the LCD as a fundamental skill in various disciplines. Understanding this concept empowers individuals to solve problems, make calculations, and analyze data involving fractions with different denominators, contributing to successful outcomes in a range of fields.
Key Insights:
| Concept | Importance |
|---|---|
| Finding the LCD is a crucial step in many applications that involve fractions with different denominators. | Enables accurate calculations, problem-solving, and analysis in various fields. |
| Understanding how to find the LCD empowers individuals to effectively solve problems and make calculations involving fractions. | Contributes to successful outcomes in cooking, chemistry, physics, and other disciplines. |
FAQs on Finding the LCD
This section addresses common questions and misconceptions surrounding the concept of finding the lowest common denominator (LCD) of fractions.
Question 1: Why is it important to find the LCD?
Finding the LCD is essential for adding, subtracting, or comparing fractions with different denominators. It provides a common denominator that allows for these operations to be performed accurately and efficiently.
Question 2: How do I find the LCD of two or more fractions?
To find the LCD, multiply the denominators of all the fractions together. The result is the LCD.
Question 3: What is the relationship between the LCD and GCF?
The LCD is always a multiple of the greatest common factor (GCF) of the numerators and denominators of the fractions. In other words, the GCF is a factor of the LCD.
Question 4: Can I use the LCD to simplify fractions?
Yes, the LCD can be used to simplify fractions by dividing both the numerator and denominator by the GCF.
Question 5: How is finding the LCD useful in real-world applications?
Finding the LCD has practical applications in various fields, including cooking, chemistry, and physics. It allows for accurate calculations and comparisons involving fractions.
Question 6: Where can I learn more about finding the LCD?
There are numerous resources available online and in libraries that provide detailed explanations and examples on finding the LCD. Consult textbooks, online tutorials, or seek guidance from a math tutor for further understanding.
Summary: Finding the LCD is a fundamental skill in mathematics that enables us to perform operations involving fractions with different denominators. It plays a crucial role in various applications, making it an important concept to grasp.
Transition to the next article section:
Tips for Finding the LCD
Finding the lowest common denominator (LCD) is a fundamental skill in mathematics. It is used to add, subtract, or compare fractions with different denominators. Here are five tips to help you find the LCD:
Tip 1: Prime Factorization
Prime factorization involves breaking down each denominator into its prime factors. The LCD is then the product of the highest powers of each unique prime factor.
Tip 2: Common Factors
Identify any common factors between the denominators. Multiply these common factors together to find a common multiple of the denominators. Continue multiplying until you have the LCD.
Tip 3: Multiples
List the multiples of each denominator. The LCD is the smallest multiple that appears in all the lists.
Tip 4: GCF and LCM
Find the greatest common factor (GCF) of the numerators and the least common multiple (LCM) of the denominators. The LCD is the product of the GCF and the LCM.
Tip 5: Inspection
For simple fractions, you may be able to find the LCD by inspection. Look for common multiples of the denominators that are also less than or equal to the numerators.
Summary:
Finding the LCD is an important skill that can be mastered with practice. By following these tips, you can improve your ability to find the LCD and solve problems involving fractions.
Transition to the article’s conclusion:
Conclusion on Finding the LCD
Finding the lowest common denominator (LCD) is a fundamental skill in mathematics that enables us to add, subtract, and compare fractions with different denominators. It is used extensively in various fields, including cooking, chemistry, and physics.
Understanding the concept of finding the LCD and developing proficiency in this skill are essential for success in mathematics and its applications. By mastering this technique, individuals can effectively solve problems, make calculations, and analyze data involving fractions, contributing to accurate outcomes and successful endeavors.
Youtube Video: