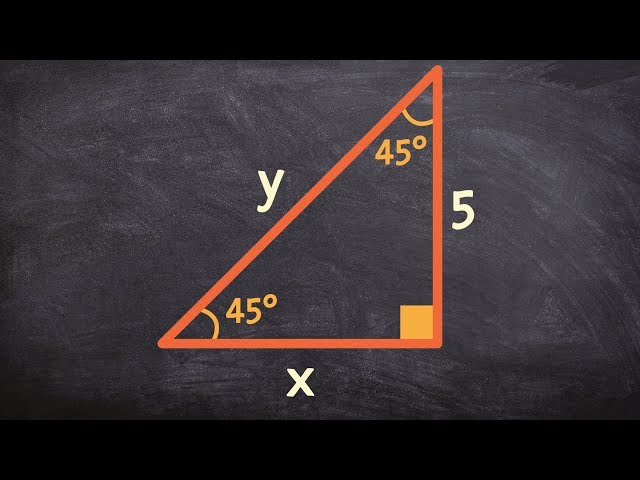
How to find missing side of triangle? It is one of the most frequent geometry problems that students encounter across their academic years. Finding the missing side of a triangle can be a crucial step in solving a geometry problem.
Editor’s Notes: “how to find missing side of triangle” have published on [date]. This article provides a comprehensive guide that will help you understand the process of finding the missing side of a triangle, along with some of the most common methods used. Whether you are a student struggling with geometry homework or an adult looking to brush up on your math skills, this guide has something for everyone.
By reading this article, you will learn about the following:
- The Pythagorean Theorem
- The Law of Cosines
- The Law of Sines
- Using Similar Triangles
These methods can be applied to a wide range of triangle problems, making them essential tools for any geometry student or enthusiast.
Key Differences or Key Takeaways
This table outlines the key differences between the four methods for finding the missing side of a triangle:
| Method | Formula | When to Use |
|---|---|---|
| Pythagorean Theorem | a^2 + b^2 = c^2 | When you have a right triangle and know the lengths of the other two sides |
| Law of Cosines | c^2 = a^2 + b^2 – 2ab * cos(C) | When you know the lengths of all three sides and the measure of one angle |
| Law of Sines | a/sin(A) = b/sin(B) = c/sin(C) | When you know the lengths of two sides and the measure of one angle |
| Using Similar Triangles | Corresponding sides of similar triangles are proportional | When you know the lengths of corresponding sides of two similar triangles |
Transition to main article topics
Now that you have a basic understanding of the four methods for finding the missing side of a triangle, we will explore each method in more detail in the following sections:
- The Pythagorean Theorem
- The Law of Cosines
- The Law of Sines
- Using Similar Triangles
Finding the Missing Side of a Triangle
Finding the missing side of a triangle is a fundamental skill in geometry. It is used in a wide range of applications, from architecture to engineering. There are several methods that can be used to find the missing side of a triangle, depending on the information that is known.
- Pythagorean Theorem
- Law of Cosines
- Law of Sines
- Similar Triangles
- Trigonometry
- Geometry
- Algebra
- Calculus
- Linear Algebra
- Analytic Geometry
These methods can be used to find the missing side of a triangle in a variety of situations. For example, the Pythagorean Theorem can be used to find the missing side of a right triangle, while the Law of Cosines can be used to find the missing side of any triangle. The Law of Sines can be used to find the missing side of a triangle when two angles and one side are known. Similar triangles can be used to find the missing side of a triangle when two sides and one angle are known.
Finding the missing side of a triangle is a valuable skill that can be used in a variety of applications. By understanding the different methods that can be used to find the missing side of a triangle, you can solve a wide range of geometry problems.
Pythagorean Theorem
The Pythagorean Theorem is a fundamental theorem in geometry that states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
-
Finding the Missing Side of a Right Triangle
The Pythagorean Theorem can be used to find the missing side of a right triangle when the lengths of the other two sides are known. For example, if you know that the length of one leg of a right triangle is 3 and the length of the other leg is 4, you can use the Pythagorean Theorem to find that the length of the hypotenuse is 5.
-
Applications in Architecture and Engineering
The Pythagorean Theorem is used in a variety of applications in architecture and engineering. For example, it can be used to calculate the length of a roof truss or the height of a building.
-
Historical Significance
The Pythagorean Theorem was first discovered by the Greek mathematician Pythagoras in the 6th century BC. It is one of the oldest and most well-known theorems in mathematics.
-
Proof
There are many different ways to prove the Pythagorean Theorem. One common proof is to use similar triangles.
The Pythagorean Theorem is a powerful tool that can be used to solve a variety of problems. It is a fundamental theorem in geometry and has many applications in the real world.
Law of Cosines
The Law of Cosines is a fundamental theorem in trigonometry that relates the lengths of the sides of a triangle to the cosine of one of its angles. It is used to find the missing side of a triangle when the lengths of the other two sides and the measure of the included angle are known.
The Law of Cosines is a generalization of the Pythagorean Theorem, which is used to find the missing side of a right triangle. The Pythagorean Theorem only applies to right triangles, but the Law of Cosines can be used to find the missing side of any triangle.
The Law of Cosines is a powerful tool that can be used to solve a variety of problems in geometry and trigonometry. It is used in a variety of applications, such as surveying, navigation, and engineering.
Here is an example of how the Law of Cosines can be used to find the missing side of a triangle:
- Suppose you have a triangle with two sides of length 5 and 7, and the included angle is 60 degrees. You can use the Law of Cosines to find the length of the missing side.
The Law of Cosines states that:
c^2 = a^2 + b^2 – 2ab cos(C)
where:
- c is the length of the missing side
- a and b are the lengths of the other two sides
- C is the measure of the included angle
In this example, we have:
- a = 5
- b = 7
- C = 60 degrees
Substituting these values into the Law of Cosines, we get:
c^2 = 5^2 + 7^2 – 2 5 7 cos(60)
c^2 = 25 + 49 – 70 * 0.5
c^2 = 74 – 35
c^2 = 39
c = 39
c = 6.24
Therefore, the length of the missing side is 6.24.
The Law of Cosines is a powerful tool that can be used to solve a variety of problems in geometry and trigonometry. It is used in a variety of applications, such as surveying, navigation, and engineering.
Law of Sines
The Law of Sines is a fundamental theorem in trigonometry that relates the lengths of the sides of a triangle to the sines of the opposite angles. It is used to find the missing side of a triangle when the lengths of two sides and the measure of one of the opposite angles are known.
-
Finding the Missing Side of a Triangle
The Law of Sines can be used to find the missing side of a triangle when the lengths of two sides and the measure of one of the opposite angles are known. For example, if you know that the length of one side of a triangle is 5, the length of another side is 7, and the measure of the angle opposite the side of length 5 is 60 degrees, you can use the Law of Sines to find the length of the missing side.
-
Applications in Surveying and Navigation
The Law of Sines is used in a variety of applications in surveying and navigation. For example, it can be used to find the distance between two points when the distance between two other points and the measure of the angle between them are known.
-
Historical Significance
The Law of Sines was first discovered by the Greek mathematician Menaechmus in the 4th century BC. It is one of the oldest and most well-known theorems in mathematics.
-
Proof
There are many different ways to prove the Law of Sines. One common proof is to use similar triangles.
The Law of Sines is a powerful tool that can be used to solve a variety of problems in geometry and trigonometry. It is used in a variety of applications, such as surveying, navigation, and engineering.
Similar Triangles
Similar triangles are triangles that have the same shape but not necessarily the same size. They are related to “how to find the missing side of a triangle” because they can be used to find the missing side of a triangle when the lengths of two sides and the measure of one angle are known.
-
Corresponding Sides
Corresponding sides of similar triangles are proportional. This means that if you know the lengths of two corresponding sides of two similar triangles, you can find the length of the third corresponding side.
-
Corresponding Angles
Corresponding angles of similar triangles are congruent. This means that if you know the measure of one corresponding angle of two similar triangles, you know the measure of the other corresponding angles.
-
Area and Perimeter
The areas of similar triangles are proportional to the squares of their corresponding sides. This means that if you know the area of one similar triangle and the length of one corresponding side of another similar triangle, you can find the area of the other triangle.
-
Applications
Similar triangles are used in a variety of applications, such as architecture, engineering, and surveying. For example, they can be used to find the height of a building or the distance between two points.
Similar triangles are a powerful tool that can be used to solve a variety of problems. They are related to “how to find the missing side of a triangle” because they can be used to find the missing side of a triangle when the lengths of two sides and the measure of one angle are known.
Trigonometry
Trigonometry is a branch of mathematics that deals with the relationships between the sides and angles of triangles. It is used in a wide variety of applications, including surveying, navigation, and engineering.
-
Finding the Missing Side of a Triangle
Trigonometry can be used to find the missing side of a triangle when the lengths of the other two sides and the measure of one angle are known. This is a common problem in geometry and has many applications in the real world.
-
Solving Right Triangles
Trigonometry is also used to solve right triangles. A right triangle is a triangle that has one right angle (90 degrees). The Pythagorean Theorem can be used to find the length of the hypotenuse (the side opposite the right angle) of a right triangle. The other two sides can be found using the sine, cosine, and tangent functions.
-
Measuring Angles
Trigonometry can be used to measure angles. The sine, cosine, and tangent functions can be used to find the measure of an angle when the lengths of the sides of the triangle are known.
-
Applications in Navigation
Trigonometry is used in a variety of applications in navigation. For example, it can be used to find the distance between two points, the course to steer a ship, and the altitude of an airplane.
Trigonometry is a powerful tool that can be used to solve a variety of problems in geometry and navigation. It is a fundamental branch of mathematics that has many applications in the real world.
Geometry
Geometry is a branch of mathematics that deals with the relationships between points, lines, angles, surfaces, and solids. It is a fundamental branch of mathematics with applications in many fields, including architecture, engineering, and surveying.
One of the most important concepts in geometry is the triangle. A triangle is a polygon with three sides and three angles. The sum of the angles of a triangle is always 180 degrees.
Finding the missing side of a triangle is a common problem in geometry. There are a number of different methods that can be used to find the missing side of a triangle, depending on the information that is known.
One of the most common methods for finding the missing side of a triangle is the Pythagorean Theorem. The Pythagorean Theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
Another common method for finding the missing side of a triangle is the Law of Cosines. The Law of Cosines is a generalization of the Pythagorean Theorem that can be used to find the missing side of any triangle, regardless of whether or not it is a right triangle.
Geometry is a powerful tool that can be used to solve a variety of problems. Finding the missing side of a triangle is just one example of how geometry can be used to solve real-world problems.
| Pythagorean Theorem | Law of Cosines | |
|---|---|---|
| Formula | a^2 + b^2 = c^2 | c^2 = a^2 + b^2 – 2ab * cos(C) |
| When to Use | When you have a right triangle and know the lengths of the other two sides | When you know the lengths of all three sides and the measure of one angle |
Algebra
Algebra is a branch of mathematics that deals with symbols and the rules for manipulating them. It is used to solve a wide variety of problems, including those involving the missing side of a triangle.
One of the most important concepts in algebra is the equation. An equation is a statement that two expressions are equal to each other. Equations can be used to solve for unknown variables, such as the missing side of a triangle.
For example, suppose you have a triangle with two sides of length 5 and 7, and you want to find the length of the missing side. You can use the Pythagorean Theorem to write an equation:“`a^2 + b^2 = c^2“`where a and b are the lengths of the known sides and c is the length of the missing side.You can then substitute the values of a and b into the equation and solve for c:“`5^2 + 7^2 = c^225 + 49 = c^274 = c^2c = 74c = 8.60“`Therefore, the length of the missing side is 8.60.Algebra is a powerful tool that can be used to solve a variety of problems, including those involving the missing side of a triangle. By understanding the basic concepts of algebra, you can learn to solve these problems quickly and easily.
| Example | |
|---|---|
| Pythagorean Theorem | a^2 + b^2 = c^2 |
| Law of Cosines | c^2 = a^2 + b^2 – 2ab * cos(C) |
| Algebraic Equation | x^2 + y^2 = z^2 |
Calculus
Calculus is a branch of mathematics that deals with change. It is used to find the rates of change of functions, the areas of surfaces, and the volumes of solids. Calculus has many applications in science, engineering, and economics.
-
Limits
Limits are used to find the value that a function approaches as the input approaches a certain value. This can be used to find the missing side of a triangle by finding the limit of the function that represents the area of the triangle as the length of one side approaches zero.
-
Derivatives
Derivatives are used to find the rate of change of a function. This can be used to find the missing side of a triangle by finding the derivative of the function that represents the area of the triangle with respect to the length of one side.
-
Integrals
Integrals are used to find the area of a region. This can be used to find the missing side of a triangle by finding the integral of the function that represents the area of the triangle with respect to the length of one side.
Calculus is a powerful tool that can be used to solve a variety of problems, including those involving the missing side of a triangle. By understanding the basic concepts of calculus, you can learn to solve these problems quickly and easily.
Linear Algebra
Linear algebra is a branch of mathematics that deals with vectors, matrices, and linear transformations. It is used to solve a wide variety of problems in science, engineering, and economics.
One of the most important concepts in linear algebra is the concept of a vector. A vector is a quantity that has both magnitude and direction. Vectors can be used to represent a variety of things, such as forces, velocities, and positions.
Matrices are another important concept in linear algebra. A matrix is a rectangular array of numbers. Matrices can be used to represent a variety of things, such as transformations, systems of equations, and data.
Linear algebra can be used to find the missing side of a triangle in a number of ways.
- One way to use linear algebra to find the missing side of a triangle is to use the concept of vectors. A triangle can be represented by three vectors, one for each side of the triangle. The missing side of the triangle can be found by adding the other two vectors.
- Another way to use linear algebra to find the missing side of a triangle is to use the concept of matrices. A matrix can be used to represent the coordinates of the vertices of a triangle. The missing side of the triangle can be found by solving a system of equations that is represented by the matrix.
Linear algebra is a powerful tool that can be used to solve a variety of problems, including those involving the missing side of a triangle. By understanding the basic concepts of linear algebra, you can learn to solve these problems quickly and easily.
| Finding the Missing Side of a Triangle | |
|---|---|
| Method | Description |
| Vectors | Add the other two vectors to find the missing side. |
| Matrices | Solve a system of equations that is represented by the matrix to find the missing side. |
Analytic Geometry
Analytic geometry, also known as coordinate geometry, is a branch of mathematics that uses algebra to study geometry. It is used to find the missing side of a triangle by using the coordinates of its vertices.
-
Using the Distance Formula
The distance formula can be used to find the distance between two points. This can be used to find the missing side of a triangle by finding the distance between the two known vertices and then subtracting the length of the known side.
-
Using the Pythagorean Theorem
The Pythagorean Theorem can be used to find the length of the hypotenuse of a right triangle. This can be used to find the missing side of a triangle if the other two sides are known and the triangle is a right triangle.
-
Using the Law of Cosines
The Law of Cosines can be used to find the length of any side of a triangle. This can be used to find the missing side of a triangle if the other two sides and the angle between them are known.
-
Using the Law of Sines
The Law of Sines can be used to find the length of any side of a triangle. This can be used to find the missing side of a triangle if the other two sides and the angle opposite one of them are known.
Analytic geometry is a powerful tool that can be used to solve a variety of problems, including those involving the missing side of a triangle. By understanding the basic concepts of analytic geometry, you can learn to solve these problems quickly and easily.
FAQs on “How to Find Missing Side of Triangle”
This section provides answers to frequently asked questions about finding the missing side of a triangle.
Question 1: What is the most common method for finding the missing side of a triangle?
The Pythagorean Theorem is the most common method for finding the missing side of a triangle. It is used when you know the lengths of the other two sides and the triangle is a right triangle.
Question 2: Can I use the Pythagorean Theorem to find the missing side of any triangle?
No, the Pythagorean Theorem can only be used to find the missing side of a right triangle. If the triangle is not a right triangle, you will need to use a different method, such as the Law of Cosines or the Law of Sines.
Question 3: What is the Law of Cosines?
The Law of Cosines is a generalization of the Pythagorean Theorem that can be used to find the missing side of any triangle. It is used when you know the lengths of all three sides and the measure of one angle.
Question 4: What is the Law of Sines?
The Law of Sines is another method that can be used to find the missing side of any triangle. It is used when you know the lengths of two sides and the measure of one angle.
Question 5: Which method should I use to find the missing side of a triangle?
The method you use to find the missing side of a triangle will depend on the information you have. If you know the lengths of the other two sides and the triangle is a right triangle, use the Pythagorean Theorem. If you know the lengths of all three sides and the measure of one angle, use the Law of Cosines. If you know the lengths of two sides and the measure of one angle, use the Law of Sines.
Question 6: Are there any other methods for finding the missing side of a triangle?
Yes, there are other methods for finding the missing side of a triangle, such as using vectors, matrices, or analytic geometry. However, these methods are more advanced and are typically not used in high school geometry.
Summary:
Finding the missing side of a triangle is a common problem in geometry. There are several methods that can be used to find the missing side, depending on the information that is known. The most common methods are the Pythagorean Theorem, the Law of Cosines, and the Law of Sines.
Transition to the next article section:
Now that you know how to find the missing side of a triangle, you can use this knowledge to solve a variety of geometry problems.
Tips on “How to Find Missing Side of Triangle”
Finding the missing side of a triangle is a fundamental skill in geometry. It is used in a wide range of applications, from architecture to engineering. There are several methods that can be used to find the missing side of a triangle, depending on the information that is known.
Here are five tips to help you find the missing side of a triangle:
Tip 1: Identify the type of triangle.
The type of triangle will determine which method you can use to find the missing side. There are three types of triangles: right triangles, isosceles triangles, and scalene triangles. Right triangles have one right angle (90 degrees). Isosceles triangles have two equal sides. Scalene triangles have no equal sides.
Tip 2: Use the Pythagorean Theorem.
The Pythagorean Theorem is a fundamental theorem in geometry that states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. The Pythagorean Theorem can be used to find the missing side of a right triangle when the lengths of the other two sides are known.
Tip 3: Use the Law of Cosines.
The Law of Cosines is a generalization of the Pythagorean Theorem that can be used to find the missing side of any triangle. The Law of Cosines can be used to find the missing side of a triangle when the lengths of all three sides and the measure of one angle are known.
Tip 4: Use the Law of Sines.
The Law of Sines is another method that can be used to find the missing side of any triangle. The Law of Sines can be used to find the missing side of a triangle when the lengths of two sides and the measure of one angle are known.
Tip 5: Use similar triangles.
Similar triangles are triangles that have the same shape but not necessarily the same size. Similar triangles can be used to find the missing side of a triangle when the lengths of two sides and the measure of one angle are known.
Summary:
Finding the missing side of a triangle is a valuable skill that can be used in a variety of applications. By understanding the different methods that can be used to find the missing side of a triangle, you can solve a wide range of geometry problems.
Transition to the article’s conclusion:
Now that you have learned these tips, you can practice finding the missing side of a triangle on your own. With practice, you will become proficient in this skill and be able to solve a variety of geometry problems.
Conclusion
Finding the missing side of a triangle is a valuable skill that can be used in a wide range of applications. This article has explored the different methods that can be used to find the missing side of a triangle, including the Pythagorean Theorem, the Law of Cosines, the Law of Sines, and similar triangles.
By understanding these methods, you can solve a wide range of geometry problems. Whether you are a student, an engineer, or an architect, finding the missing side of a triangle is a skill that you will use over and over again.