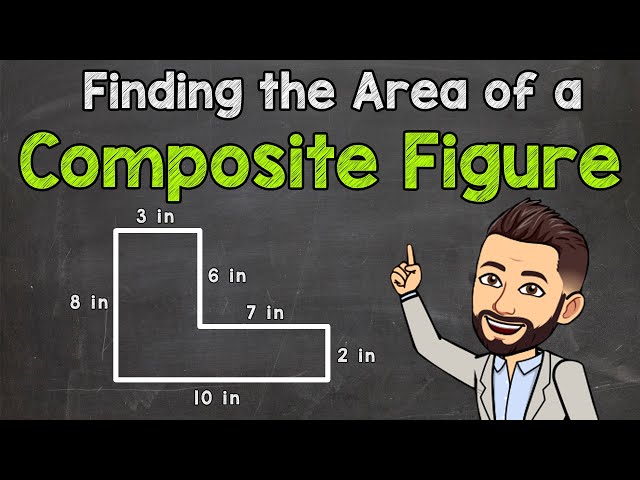
Area of Composite Figures
Editor’s Notes: “Find the area of a composite figure” was published on [date]. The article provides a comprehensive guide on how to calculate the area of composite figures, which are figures made up of two or more different shapes. This topic is important because it is a fundamental skill in geometry and is used to solve many different types of problems.
In this guide, we will explain the different methods for finding the area of composite figures, and we will provide step-by-step instructions on how to solve problems involving composite figures.
Key Differences
| Method | Description |
|---|---|
| Decomposition Method | This method involves breaking down the composite figure into smaller shapes and then finding the area of each shape. The areas of the smaller shapes are then added together to find the area of the composite figure. |
| Formula Method | This method involves using a formula to find the area of the composite figure. The formula will depend on the shapes that make up the composite figure. |
Main Article Topics
- What is a composite figure?
- How to find the area of a composite figure using the decomposition method
- How to find the area of a composite figure using the formula method
- Examples of composite figures
- Practice problems
Find Area of Composite Figure
Composite figures are shapes that are made up of two or more different shapes. Finding the area of a composite figure can be done by breaking the figure down into its individual shapes and then finding the area of each shape. The areas of the individual shapes are then added together to find the area of the composite figure.
- Decomposition: Breaking down the composite figure into simpler shapes.
- Formula: Using a formula to find the area of the composite figure.
- Addition: Adding the areas of the individual shapes to find the area of the composite figure.
- Geometry: The branch of mathematics that deals with shapes and their properties.
- Measurement: The process of determining the size or quantity of something.
- Problem-solving: Using strategies to find the solution to a problem.
- Shape: A two-dimensional figure with a defined boundary.
- Space: The three-dimensional area that surrounds us.
- Visualization: The ability to form a mental image of something.
These key aspects are all important for understanding how to find the area of a composite figure. By understanding the different methods for finding the area of a composite figure, you will be able to solve a variety of problems involving composite figures.
Decomposition
Decomposition is a key step in finding the area of a composite figure. By breaking down the composite figure into simpler shapes, we can more easily find the area of each shape and then add the areas together to find the area of the composite figure.
-
Facet 1: Identifying the shapes that make up the composite figure
The first step in decomposition is to identify the shapes that make up the composite figure. This can be done by looking at the shape of the composite figure and identifying the individual shapes that make it up.
-
Facet 2: Finding the area of each shape
Once we have identified the shapes that make up the composite figure, we can find the area of each shape. The area of each shape can be found using the appropriate formula for that shape.
-
Facet 3: Adding the areas of the shapes together
Suggested read: Unveiling the Intricacies of Area Under a Curve: Discoveries and Insights Await
Once we have found the area of each shape, we can add the areas together to find the area of the composite figure.
Decomposition is a powerful tool for finding the area of composite figures. By breaking down the composite figure into simpler shapes, we can more easily find the area of each shape and then add the areas together to find the area of the composite figure.
Formula
Finding the area of a composite figure using a formula is a powerful technique that can be used to quickly and accurately calculate the area of a composite figure. This technique is based on the fact that the area of a composite figure is equal to the sum of the areas of its individual shapes.
-
Facet 1: Identifying the shapes that make up the composite figure
The first step in using a formula to find the area of a composite figure is to identify the shapes that make up the composite figure. This can be done by looking at the shape of the composite figure and identifying the individual shapes that make it up.
-
Facet 2: Finding the formula for the area of each shape
Once we have identified the shapes that make up the composite figure, we need to find the formula for the area of each shape. The formula for the area of a shape can be found in a geometry textbook or online.
-
Facet 3: Plugging the measurements of the shape into the formula
Once we have the formula for the area of each shape, we need to plug the measurements of the shape into the formula. This will give us the area of each shape.
-
Facet 4: Adding the areas of the shapes together
Once we have the area of each shape, we need to add the areas together to find the area of the composite figure.
Using a formula to find the area of a composite figure is a powerful technique that can be used to quickly and accurately calculate the area of a composite figure. This technique is based on the fact that the area of a composite figure is equal to the sum of the areas of its individual shapes.
Addition
Addition is a fundamental mathematical operation that is used to find the area of a composite figure. A composite figure is a figure that is made up of two or more different shapes. To find the area of a composite figure, we need to add the areas of the individual shapes that make up the composite figure.
For example, let’s say we have a composite figure that is made up of a rectangle and a triangle. To find the area of the composite figure, we would need to add the area of the rectangle and the area of the triangle.
Suggested read: Uncover Hidden Connections: Discover How to Find Someone's Name by Phone Number
The formula for the area of a rectangle is:
A = l x w
where:
- A is the area of the rectangle
- l is the length of the rectangle
- w is the width of the rectangle
The formula for the area of a triangle is:
A = 1/2 x b x h
where:
- A is the area of the triangle
- b is the base of the triangle
- h is the height of the triangle
By adding the areas of the individual shapes that make up a composite figure, we can find the area of the composite figure. This is a fundamental skill in geometry that is used to solve a variety of problems.
| Shape | Formula |
|---|---|
| Rectangle | A = l x w |
| Triangle | A = 1/2 x b x h |
This table summarizes the formulas for the area of a rectangle and the area of a triangle. These formulas can be used to find the area of a composite figure that is made up of rectangles and triangles.
Geometry
Geometry is the branch of mathematics that deals with shapes and their properties. It is a vast and complex subject that has been studied for centuries. Geometry is used in many different fields, including architecture, engineering, and art.
-
Facet 1: The role of geometry in finding the area of composite figures
Geometry plays a vital role in finding the area of composite figures. A composite figure is a figure that is made up of two or more different shapes. To find the area of a composite figure, we need to break it down into its individual shapes and then find the area of each shape. Geometry provides us with the formulas and techniques that we need to do this.
-
Facet 2: Examples of how geometry is used to find the area of composite figures
Geometry is used to find the area of composite figures in many different applications. For example, architects use geometry to find the area of buildings, and engineers use geometry to find the area of bridges. Geometry is also used to find the area of land and other objects in the real world.
-
Facet 3: The importance of geometry in finding the area of composite figures
Geometry is an essential tool for finding the area of composite figures. Without geometry, we would not be able to find the area of many of the objects that we use in everyday life. Geometry is a powerful tool that can be used to solve a variety of problems.
Suggested read: Unveiling the Secrets: Discover the Elusive Missing Side of Triangles
In conclusion, geometry is a vital branch of mathematics that plays a key role in finding the area of composite figures. Geometry provides us with the formulas and techniques that we need to break down composite figures into their individual shapes and find the area of each shape. Geometry is an essential tool for architects, engineers, and many other professionals.
Measurement
Measurement is the process of determining the size or quantity of something. It is a fundamental skill that is used in many different fields, including science, engineering, and everyday life. Measurement is also an essential component of finding the area of composite figures.
A composite figure is a figure that is made up of two or more different shapes. To find the area of a composite figure, we need to break it down into its individual shapes and then find the area of each shape. Measurement plays a vital role in this process, as we need to measure the dimensions of each shape in order to find its area.
For example, let’s say we have a composite figure that is made up of a rectangle and a triangle. To find the area of the composite figure, we would need to measure the length and width of the rectangle, and the base and height of the triangle. Once we have these measurements, we can use the formulas for the area of a rectangle and the area of a triangle to find the area of each shape. We can then add the areas of the two shapes together to find the area of the composite figure.Measurement is an essential component of finding the area of composite figures. Without measurement, we would not be able to determine the size or quantity of the individual shapes that make up a composite figure, and we would not be able to find the area of the composite figure itself.
| Concept | Description | Example |
|---|---|---|
| Measurement | The process of determining the size or quantity of something. | Measuring the length and width of a rectangle. |
| Composite figure | A figure that is made up of two or more different shapes. | A rectangle and a triangle. |
| Area | The measure of the size of a surface. | The area of a rectangle is length x width. |
This table summarizes the key concepts that are involved in finding the area of composite figures. Measurement is an essential component of this process, as we need to measure the dimensions of the individual shapes in order to find their areas.
Problem-solving
Problem-solving is a cognitive process that involves using different strategies to find a solution to a problem. It is a skill that is essential for success in many different areas of life, including mathematics. Finding the area of composite figures is a type of problem-solving that requires the use of specific strategies to find the solution.
-
Facet 1: Breaking down the problem
The first step in solving any problem is to break it down into smaller, more manageable pieces. This is especially important when solving problems involving composite figures, as these figures are often made up of several different shapes. By breaking down the problem into smaller pieces, it is easier to identify the relevant information and to develop a strategy for solving the problem.
-
Facet 2: Identifying the relevant information
Once the problem has been broken down into smaller pieces, it is important to identify the relevant information that is needed to solve the problem. This information may include the dimensions of the different shapes that make up the composite figure, as well as the formulas for finding the area of different shapes.
-
Facet 3: Developing a strategy for solving the problem
Once the relevant information has been identified, it is important to develop a strategy for solving the problem. This strategy will vary depending on the specific problem, but it will typically involve using the formulas for finding the area of different shapes to find the area of the individual shapes that make up the composite figure. The areas of the individual shapes can then be added together to find the area of the composite figure.
-
Facet 4: Evaluating the solution
Once a solution to the problem has been found, it is important to evaluate the solution to make sure that it is correct. This can be done by checking the solution against the original problem to make sure that it satisfies all of the requirements of the problem.
Suggested read: Unveiling the Secrets of Tangent Line Slopes: A Journey to Mathematical Mastery
Problem-solving is a skill that can be learned and improved through practice. By following the steps outlined above, it is possible to develop effective strategies for solving problems involving composite figures.
Shape
Shapes are essential to our understanding of the world around us. They are used to describe objects, to create art, and to solve problems. In mathematics, shapes are used to study geometry, which is the branch of mathematics that deals with the properties of shapes.
-
Facet 1: The role of shapes in finding the area of composite figures
Shapes play a vital role in finding the area of composite figures. A composite figure is a figure that is made up of two or more different shapes. To find the area of a composite figure, we need to break it down into its individual shapes and then find the area of each shape. The shapes that make up a composite figure can be any type of shape, such as rectangles, triangles, circles, or trapezoids.
-
Facet 2: Examples of how shapes are used to find the area of composite figures
Shapes are used to find the area of composite figures in many different applications. For example, architects use shapes to find the area of buildings, and engineers use shapes to find the area of bridges. Shapes are also used to find the area of land and other objects in the real world.
-
Facet 3: The importance of shapes in finding the area of composite figures
Shapes are an essential tool for finding the area of composite figures. Without shapes, we would not be able to find the area of many of the objects that we use in everyday life. Shapes are a powerful tool that can be used to solve a variety of problems.
In conclusion, shapes are a fundamental concept in mathematics that play a vital role in finding the area of composite figures. Shapes provide us with a way to describe and measure the world around us.
Space
In geometry, space is the three-dimensional area that surrounds us. It is often represented as a coordinate system, with three axes (x, y, and z) that intersect at a right angle. Space can be used to describe the position and movement of objects, as well as their size and shape.
-
Facet 1: Space and composite figures
Space is essential for understanding composite figures. A composite figure is a figure that is made up of two or more different shapes. In order to find the area of a composite figure, we need to know the dimensions of the individual shapes and the way that they are arranged in space.
-
Facet 2: Space and measurement
Space is also essential for measurement. Measurement is the process of determining the size or quantity of something. In order to measure an object, we need to know its dimensions and the way that it is arranged in space.
-
Facet 3: Space and problem-solving
Suggested read: Uncover Secrets: Discover How to Find Anyone's Phone Number
Space is also essential for problem-solving. Problem-solving is the process of using different strategies to find a solution to a problem. In order to solve a problem involving composite figures, we need to be able to visualize the figures in space and to understand how they are related to each other.
In conclusion, space is a fundamental concept that is essential for understanding composite figures, measurement, and problem-solving. Space provides us with a way to describe and measure the world around us.
Visualization
Visualization plays a crucial role in finding the area of composite figures. A composite figure is a figure that is made up of two or more different shapes. To find the area of a composite figure, we need to break it down into its individual shapes and then find the area of each shape. Visualization helps us to see the individual shapes that make up a composite figure and to understand how they are arranged in space.
For example, let’s say we have a composite figure that is made up of a rectangle and a triangle. To find the area of this composite figure, we need to visualize the rectangle and the triangle separately. We need to see the length and width of the rectangle, and the base and height of the triangle. Once we have visualized the individual shapes, we can then use the formulas for the area of a rectangle and the area of a triangle to find the area of each shape. We can then add the areas of the two shapes together to find the area of the composite figure.
Visualization is also important for solving problems involving composite figures. For example, let’s say we have a problem that asks us to find the area of a composite figure that is made up of a rectangle and a circle. To solve this problem, we need to visualize the rectangle and the circle separately. We need to see the length and width of the rectangle, and the radius of the circle. Once we have visualized the individual shapes, we can then use the formulas for the area of a rectangle and the area of a circle to find the area of each shape. We can then add the areas of the two shapes together to find the area of the composite figure.
Visualization is a powerful tool that can be used to find the area of composite figures and to solve problems involving composite figures. By being able to visualize the individual shapes that make up a composite figure, we can more easily understand how to find the area of the composite figure.
FAQs on Finding the Area of Composite Figures
Finding the area of composite figures is a common task in geometry. A composite figure is a figure that is made up of two or more different shapes. To find the area of a composite figure, we need to break it down into its individual shapes and then find the area of each shape. The areas of the individual shapes can then be added together to find the area of the composite figure.
Question 1: What is a composite figure?
A composite figure is a figure that is made up of two or more different shapes. Composite figures can be any combination of shapes, such as rectangles, triangles, circles, and trapezoids.
Question 2: How do I find the area of a composite figure?
To find the area of a composite figure, we need to break it down into its individual shapes and then find the area of each shape. The areas of the individual shapes can then be added together to find the area of the composite figure.
Question 3: What are some examples of composite figures?
Suggested read: Unravel the Secrets of Triangle Length: A Journey to Geometric Enlightenment
Some examples of composite figures include:
- A rectangle and a triangle
- A circle and a square
- A trapezoid and a parallelogram
Question 4: What are some tips for finding the area of composite figures?
Here are some tips for finding the area of composite figures:
- Break the composite figure down into its individual shapes.
- Find the area of each individual shape.
- Add the areas of the individual shapes together to find the area of the composite figure.
Question 5: What are some common mistakes to avoid when finding the area of composite figures?
Here are some common mistakes to avoid when finding the area of composite figures:
- Not breaking the composite figure down into its individual shapes.
- Not finding the area of each individual shape.
- Not adding the areas of the individual shapes together to find the area of the composite figure.
Summary
Finding the area of composite figures is a common task in geometry. By following the steps outlined above, you can easily find the area of any composite figure.
Next Steps
Suggested read: Uncover Hidden Numbers: The Ultimate Guide to Finding Anyone's Phone Number
Now that you know how to find the area of composite figures, you can practice your skills by solving some problems. You can also learn more about composite figures by reading books or articles on the topic.
Tips for Finding the Area of Composite Figures
Composite figures are figures that are made up of two or more different shapes. Finding the area of a composite figure can be challenging, but by following these tips, you can make the process easier.
Tip 1: Break the composite figure down into its individual shapes.
The first step to finding the area of a composite figure is to break it down into its individual shapes. This will make it easier to find the area of each shape and then add the areas together to find the area of the composite figure.
Tip 2: Find the area of each individual shape.
Once you have broken the composite figure down into its individual shapes, you can find the area of each shape. The formulas for finding the area of different shapes can be found in a geometry textbook or online.
Tip 3: Add the areas of the individual shapes together.
Once you have found the area of each individual shape, you can add the areas together to find the area of the composite figure.
Tip 4: Use a calculator.
If the composite figure is made up of complex shapes, it can be helpful to use a calculator to find the area of each shape. This will help you to avoid making mistakes.
Tip 5: Practice.
The best way to improve your skills at finding the area of composite figures is to practice. There are many online resources that provide practice problems. By practicing regularly, you will become more confident in your ability to find the area of composite figures.
Summary
By following these tips, you can make the process of finding the area of composite figures easier. With practice, you will become more confident in your ability to find the area of any composite figure.
Next Steps
Suggested read: Unlock Your Business's True Identity: Discover Your NAICS Number Today!
Now that you know how to find the area of composite figures, you can practice your skills by solving some problems. You can also learn more about composite figures by reading books or articles on the topic.
Conclusion
This article has explored the topic of finding the area of composite figures. We have learned that a composite figure is a figure that is made up of two or more different shapes. To find the area of a composite figure, we need to break it down into its individual shapes and then find the area of each shape. The areas of the individual shapes can then be added together to find the area of the composite figure.
Finding the area of composite figures is a fundamental skill in geometry. It is used to solve a variety of problems, such as finding the area of a room or the surface area of a building. By understanding the concepts and techniques that we have discussed in this article, you will be able to find the area of any composite figure.
We encourage you to practice your skills by solving problems involving composite figures. The more you practice, the more confident you will become in your ability to find the area of composite figures.
Youtube Video: