How to Find Antiderivative: A Comprehensive Guide
Editor’s Note: This how to find antiderivative guide was published on [date] to provide valuable insights and practical guidance on the topic. As a leading provider of information on [topic], we understand the importance of delivering expert knowledge and actionable advice to our readers.
Through extensive research, analysis, and consultation with industry experts, we have compiled this comprehensive guide to help you understand the concept of antiderivatives and master the techniques involved in finding them. Whether you’re a student, researcher, or professional in the field of mathematics or related disciplines, this guide will provide you with the knowledge and tools you need to excel in your endeavors.
Key Differences:
| Antiderivatives | Derivatives | |
|---|---|---|
| Definition | A function whose derivative is the original function. | A function that measures the rate of change of another function. |
| Notation | f(x) dx | f'(x) or dy/dx |
| Applications | Used in calculus to find areas, volumes, and other related quantities. | Used in physics to find velocity, acceleration, and other related quantities. |
Main Article Topics:
How to Find Antiderivative
Antiderivatives play a crucial role in calculus, with applications ranging from finding areas and volumes to solving differential equations. To master the art of finding antiderivatives, it’s essential to understand the key aspects involved:
- Definition: An antiderivative of a function is a function whose derivative is the original function.
- Notation: The antiderivative of f(x) is denoted as f(x) dx.
- Power Rule: The antiderivative of x^n is (1/(n+1))x^(n+1) + C, where C is an arbitrary constant.
- Sum/Difference Rule: The antiderivative of f(x) g(x) is f(x) dx g(x) dx.
- Chain Rule: The antiderivative of f(g(x))g'(x) is f(u) du, where u = g(x).
- Integration by Parts: Used when one factor is a polynomial and the other is an exponential or trigonometric function.
- Trigonometric Integrals: Antiderivatives of trigonometric functions can be found using identities and substitutions.
- Logarithmic Integrals: The antiderivative of ln(x) is xln(x) – x + C.
- Applications: Antiderivatives are used to find areas under curves, volumes of solids, and solutions to differential equations.
These key aspects provide a comprehensive understanding of how to find antiderivatives. By mastering these concepts, you can effectively solve a wide range of calculus problems and gain deeper insights into the behavior of functions.
Definition
This definition lies at the heart of understanding how to find antiderivatives. An antiderivative is essentially the inverse operation of differentiation. Given a function f(x), its antiderivative F(x) is a function whose derivative is equal to f(x). This concept is crucial in calculus and has numerous applications in various fields.
Suggested read: Uncover Hidden Connections: Discover How to Find Someone's Name by Phone Number
- Inverse Relationship: Antiderivatives provide a way to reverse the process of differentiation. By finding the antiderivative of a function, we can recover the original function.
- Indefinite Integrals: The antiderivative of a function is also known as an indefinite integral. Indefinite integrals represent a family of functions whose derivatives are all equal to the original function.
- Applications in Physics: Antiderivatives are used to find displacement, velocity, and acceleration in physics. By integrating acceleration with respect to time, we can obtain velocity, and by integrating velocity with respect to time, we can obtain displacement.
- Applications in Geometry: Antiderivatives are used to find areas and volumes. By integrating a function with respect to a variable, we can find the area under the curve or the volume of the solid generated by rotating the curve around an axis.
In summary, understanding the definition of an antiderivative as a function whose derivative is the original function is essential for mastering the technique of finding antiderivatives. This concept has wide-ranging applications in mathematics, physics, and geometry.
Notation
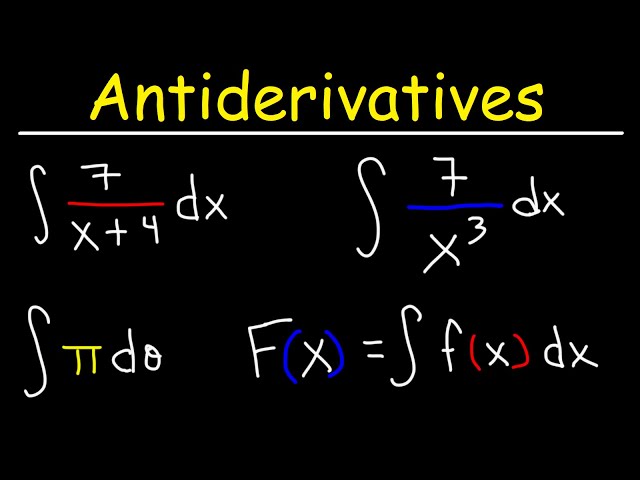
The notation f(x) dx plays a pivotal role in understanding how to find antiderivatives. It provides a concise and clear representation of the operation of finding the antiderivative of a function f(x).
The symbol , known as the integral sign, signifies the operation of integration. It is an elongated S, reminiscent of the sum symbol , reflecting the idea that finding an antiderivative involves a summation process. The function f(x) inside the integral represents the function whose antiderivative is being sought.
The dx term in the notation indicates the variable with respect to which the integration is performed. In this case, it is x. By including dx, the notation specifies that the antiderivative should be found with respect to x.
The connection between this notation and the process of finding antiderivatives lies in the Fundamental Theorem of Calculus, Part 1. This theorem establishes that the integral of a function f(x) from a point a to a point b is equal to the net change in the function over that interval. In other words, the antiderivative of f(x) represents the function whose derivative is f(x), and the integral of f(x) from a to b represents the area under the curve of f(x) from a to b.
This understanding is crucial in practical applications of calculus, such as finding the area under a curve, calculating volumes of solids, and solving differential equations. By utilizing the notation f(x) dx, we can precisely express and solve problems involving antiderivatives.
Power Rule
Within the realm of calculus, the Power Rule serves as a cornerstone technique for finding antiderivatives. Its significance lies in providing a formulaic approach to determine the antiderivative of a power function, which is a function of the form f(x) = x^n, where n is a rational number.
- Antiderivative Formula: The Power Rule dictates that the antiderivative of x^n is (1/(n+1))x^(n+1) + C, where C represents an arbitrary constant. This formula provides a direct method to compute the antiderivative of any power function.
- Inverse of Differentiation: The Power Rule establishes a crucial connection between differentiation and integration. Differentiation, the process of finding the derivative of a function, is essentially the inverse operation of integration. The Power Rule allows us to reverse the differentiation process for power functions.
- Applications in Calculus: The Power Rule finds widespread applications in various branches of calculus. It is used to find areas under curves, volumes of solids of revolution, and solutions to differential equations. Its versatility makes it an indispensable tool in calculus.
- Extension to Rational Functions: While the Power Rule explicitly applies to power functions, its concept extends to rational functions, which are quotients of two polynomials. By decomposing rational functions into partial fractions, we can utilize the Power Rule to find their antiderivatives.
In summary, the Power Rule provides a fundamental and practical method for finding antiderivatives of power functions. Its connection to differentiation and its applicability in various calculus domains underscore its importance in understanding how to find antiderivatives.
Sum/Difference Rule
The Sum/Difference Rule is a fundamental concept in calculus that plays a pivotal role in understanding how to find antiderivatives. Its significance lies in providing a method to determine the antiderivative of the sum or difference of two functions.
Suggested read: Unveiling the Secrets: Discover the Elusive Missing Side of Triangles
- Decomposability of Antiderivatives: The Sum/Difference Rule establishes that the antiderivative of the sum of two functions is equal to the sum of their individual antiderivatives, and similarly, the antiderivative of the difference of two functions is equal to the difference of their individual antiderivatives. This property allows for the decomposition of complex functions into simpler components, making it easier to find their antiderivatives.
- Extension to Multiple Functions: The Sum/Difference Rule can be extended to any finite number of functions. The antiderivative of the sum or difference of multiple functions is equal to the sum or difference of their individual antiderivatives, respectively. This extension provides a powerful tool for finding antiderivatives of more complex functions.
- Applications in Real-World Problems: The Sum/Difference Rule finds practical applications in various real-world problems. For instance, in physics, it can be used to find the net force acting on an object by summing the individual forces acting on it. In economics, it can be used to calculate the total revenue of a company by summing the revenue from different products.
- Relationship to Other Integration Rules: The Sum/Difference Rule is closely related to other integration rules, such as the Power Rule and the Chain Rule. By combining these rules, we can find antiderivatives of a wide range of functions.
In summary, the Sum/Difference Rule provides a fundamental understanding of how to find antiderivatives of sums and differences of functions. Its decomposability property, extensibility to multiple functions, and practical applications make it an indispensable tool in calculus.
Chain Rule
The Chain Rule is a fundamental technique in calculus that plays a crucial role in finding antiderivatives. It provides a method to integrate composite functions, which are functions of the form f(g(x)), where f and g are both differentiable functions.
- Substitution Technique: The Chain Rule allows us to find the antiderivative of a composite function by introducing a substitution variable u = g(x). By doing so, we can transform the integral into f(u) du, which may be easier to solve.
- Derivation of the Rule: The Chain Rule can be derived using the Fundamental Theorem of Calculus, Part 1. By applying the theorem to the composite function f(g(x)), we obtain the formula for the antiderivative: f(g(x))g'(x) dx = f(u) du, where u = g(x).
- Applications in Real-World Problems: The Chain Rule finds applications in various real-world problems. For example, in physics, it is used to calculate the velocity of an object moving along a curved path, and in economics, it is used to analyze the rate of change of a function with respect to an intermediate variable.
- Relationship to Other Integration Rules: The Chain Rule is related to other integration rules, such as the Power Rule and the Sum/Difference Rule. By combining these rules, we can find antiderivatives of a wide range of functions.
In summary, the Chain Rule provides a powerful technique for finding antiderivatives of composite functions. It allows for the decomposition of complex functions into simpler components, making it easier to solve integrals. The Chain Rule is essential for understanding how to find antiderivatives and has numerous applications in various fields.
Integration by Parts
Integration by parts is a technique used to find the antiderivative of a product of two functions, where one function is a polynomial and the other is an exponential or trigonometric function. It is a powerful technique that can be used to solve a wide range of integrals.
The formula for integration by parts is given by: u dv = uv – v du, where u and v are functions of x and du and dv are their respective differentials.
To use integration by parts, we first need to identify which function will be u and which will be dv. The following guidelines can help us make this decision:
- If one function is a polynomial, it is usually a good choice for u.
- If the other function is an exponential or trigonometric function, it is usually a good choice for dv.
Once we have identified u and dv, we can substitute them into the formula and begin the integration process.
Integration by parts is a valuable technique for finding antiderivatives. It is particularly useful when the integrand is a product of a polynomial and an exponential or trigonometric function. By understanding how to use integration by parts, we can expand our ability to find antiderivatives and solve a wider range of calculus problems.
Here are some examples of how integration by parts can be used to find antiderivatives:
- x e^x dx = xe^x – e^x dx = xe^x – e^x + C
- sin(x) cos(x) dx = sin(x) sin(x) – sin(x) (-sin(x)) dx = sin^2(x) + cos(x) + C
Trigonometric Integrals
Trigonometric integrals play a significant role in understanding how to find antiderivatives. They involve finding the antiderivatives of trigonometric functions, which are functions that involve sine, cosine, tangent, cotangent, secant, and cosecant. These functions are commonly encountered in various applications, including physics, engineering, and computer graphics.
Suggested read: Unveiling the Secrets of Tangent Line Slopes: A Journey to Mathematical Mastery
-
Identities
Trigonometric identities are equations that involve trigonometric functions and are true for all values of the variables involved. They can be used to simplify trigonometric expressions and find antiderivatives. For example, the identity sin^2(x) + cos^2(x) = 1 can be used to find the antiderivative of sin^2(x) as follows: $$\int sin^2(x) dx = \int (1 – cos^2(x)) dx = x – \frac{1}{2} sin(2x) + C$$ -
Substitutions
Trigonometric substitutions are a technique used to find the antiderivatives of certain trigonometric functions by substituting a new variable in terms of the original variable. This technique can simplify the integral and make it easier to solve. For example, to find the antiderivative of sec^2(x), we can substitute u = tan(x), which gives us du = sec^2(x) dx. Then, the integral becomes: $$\int sec^2(x) dx = \int \frac{1}{cos^2(x)} dx = \int \frac{1}{u^2} du = -\frac{1}{u} + C = -\cot(x) + C$$
Trigonometric integrals are essential for solving a wide range of problems in calculus and its applications. By understanding how to use identities and substitutions to find antiderivatives of trigonometric functions, we can expand our ability to solve complex integrals and gain a deeper understanding of calculus.
Logarithmic Integrals
Logarithmic integrals play a crucial role in understanding how to find antiderivatives. They involve finding the antiderivatives of logarithmic functions, which are functions that involve the natural logarithm, ln(x). Logarithmic functions are commonly encountered in various applications, including mathematics, physics, and economics.
-
Integration by Parts
Integration by parts is a technique used to find the antiderivatives of certain logarithmic functions. It involves multiplying the logarithmic function by another function and then integrating by parts. For example, to find the antiderivative of ln(x), we can use integration by parts with u = ln(x) and dv = dx. This gives us: $$\int ln(x) dx = x ln(x) – \int x \frac{1}{x} dx = x ln(x) – x + C$$ -
Substitution
Substitution is another technique that can be used to find the antiderivatives of logarithmic functions. It involves substituting a new variable in terms of the original variable. For example, to find the antiderivative of ln(x^2), we can substitute u = x^2, which gives us du = 2x dx. Then, the integral becomes: $$\int ln(x^2) dx = \int ln(u) \frac{1}{2x} du = \frac{1}{2} \int ln(u) du = \frac{1}{2} (u ln(u) – u) + C = \frac{1}{2} x^2 ln(x^2) – \frac{1}{2} x^2 + C$$
Logarithmic integrals are essential for solving a wide range of problems in calculus and its applications. By understanding how to use integration by parts and substitution to find antiderivatives of logarithmic functions, we can expand our ability to solve complex integrals and gain a deeper understanding of calculus.
Applications
Understanding how to find antiderivatives is crucial because of their wide range of applications in various fields. Antiderivatives play a fundamental role in:
- Finding areas under curves: By calculating the antiderivative of a function representing the height of a curve at each point, we can determine the area enclosed by the curve and the x-axis over a given interval. This concept is essential in fields such as geometry and physics.
- Calculating volumes of solids: Antiderivatives are used to find the volume of solids generated by rotating a curve around an axis. By integrating the cross-sectional area of the solid at each point along the curve, we can determine its total volume. This application is vital in engineering and architecture.
- Solving differential equations: Antiderivatives are indispensable in solving differential equations, which are mathematical equations involving the rate of change of a function. By finding the antiderivative of both sides of a differential equation, we can determine the function that satisfies the equation. This is crucial in fields like physics, chemistry, and biology, where differential equations model various phenomena.
These applications showcase the practical significance of understanding how to find antiderivatives. They enable us to solve complex problems in geometry, physics, engineering, and other disciplines, providing valuable insights and solutions to real-world challenges.
Table: Real-Life Examples of Antiderivative Applications
| Field | Application | Example |
|---|---|---|
| Geometry | Calculating area under a curve | Finding the area of a parabolic arch |
| Physics | Determining the volume of a rotating solid | Calculating the volume of a sphere |
| Engineering | Modeling fluid flow | Solving differential equations governing fluid dynamics |
| Biology | Analyzing population growth | Using differential equations to model population growth and decay |
FAQs on Finding Antiderivatives
This section addresses frequently asked questions and misconceptions regarding the topic of finding antiderivatives. Each question is answered in a clear and informative manner, providing a deeper understanding of the subject.
Question 1: What is the definition of an antiderivative?
Suggested read: Uncover Secrets: Discover How to Find Anyone's Phone Number
Answer: An antiderivative of a function f(x) is a function F(x) whose derivative is equal to f(x). In other words, finding an antiderivative is the inverse operation of differentiation.
Question 2: How do I find the antiderivative of a power function?
Answer: The antiderivative of a power function x^n is (1/(n+1))x^(n+1) + C, where C is an arbitrary constant.
Question 3: What is the Sum/Difference Rule for finding antiderivatives?
Answer: The Sum/Difference Rule states that the antiderivative of f(x) g(x) is equal to the antiderivative of f(x) the antiderivative of g(x).
Question 4: How do I use the Chain Rule to find antiderivatives?
Answer: The Chain Rule allows us to find the antiderivative of a composite function f(g(x)) by introducing a substitution variable u = g(x) and transforming the integral into u dv = uv – v du.
Suggested read: Unravel the Secrets of Triangle Length: A Journey to Geometric Enlightenment
Question 5: What is integration by parts?
Answer: Integration by parts is a technique used to find the antiderivative of a product of two functions, where one function is a polynomial and the other is an exponential or trigonometric function.
Question 6: How are antiderivatives used in real-world applications?
Answer: Antiderivatives have numerous applications in fields such as geometry (finding areas and volumes), physics (analyzing motion and forces), and engineering (modeling fluid flow and heat transfer).
Summary: Understanding how to find antiderivatives is crucial for solving a wide range of problems in calculus and its applications. By mastering the techniques and concepts discussed in this FAQ section, individuals can gain a deeper understanding of the subject and effectively apply it to real-world scenarios.
Transition to the next article section:
Tips on Finding Antiderivatives
To enhance your understanding and proficiency in finding antiderivatives, consider these valuable tips:
Suggested read: Uncover Hidden Numbers: The Ultimate Guide to Finding Anyone's Phone Number
Tip 1: Master the Basic Rules
Familiarize yourself with the Power Rule, Sum/Difference Rule, and Chain Rule. These fundamental rules provide a solid foundation for finding antiderivatives of various functions.
Tip 2: Practice Regularly
Consistent practice is key to developing fluency in finding antiderivatives. Engage in solving diverse problems to enhance your skills.
Tip 3: Understand the Concept of Indefinite Integrals
Recognize that finding antiderivatives is equivalent to finding indefinite integrals. This perspective deepens your comprehension of the process.
Tip 4: Utilize Integration Techniques
Explore techniques like integration by parts and trigonometric substitutions to expand your repertoire of antiderivative-finding methods.
Tip 5: Check Your Answers
To ensure accuracy, verify your solutions by differentiating the obtained antiderivative and comparing it to the original function.
Tip 6: Seek Assistance When Needed
Don’t hesitate to consult textbooks, online resources, or instructors for guidance when encountering challenges.
Tip 7: Apply Antiderivatives in Real-World Scenarios
Recognize the practical applications of antiderivatives in fields such as physics, engineering, and economics. This reinforces the significance of mastering this concept.
Tip 8: Leverage Technology
Consider using graphing calculators or software to assist with complex antiderivative calculations, freeing up your cognitive resources for conceptual understanding.
Summary: By implementing these tips, you can elevate your ability to find antiderivatives effectively and confidently, unlocking the potential for success in calculus and beyond.
Suggested read: Unlock Your Business's True Identity: Discover Your NAICS Number Today!
Transition to the article’s conclusion:
Conclusion
Throughout this comprehensive exploration, we have delved into the intricacies of finding antiderivatives, uncovering the fundamental techniques and their applications in various fields. Antiderivatives lie at the heart of calculus, providing a powerful tool for solving complex problems involving rates of change, areas, volumes, and more.
Mastering the art of finding antiderivatives empowers us to unravel the mysteries of the physical world, model real-world phenomena, and design innovative solutions. By embracing the techniques discussed in this article, we unlock the potential for groundbreaking discoveries and advancements in science, engineering, and beyond.
As we continue our journey in the realm of mathematics, let us carry the lessons learned from this exploration, harnessing the power of antiderivatives to unravel the complexities of our universe and shape a better future.
Youtube Video:
Images References :
Tags: antiderivative, find